Hill Sphere: Discover the Gravitational Realm of a Celestial Body
Embark on a celestial odyssey with us as we uncover the secrets of Hill spheres—a gravitational realm that influences the dance of celestial bodies.
In this exploration, we’ll unravel the mysteries, understand celestial orbits, and reveal practical applications that stretch beyond our solar system. Join us as we navigate the cosmic depths, where gravity’s influence paints a celestial tapestry both within and beyond the enigmatic Hill sphere.
What Is a Hill Sphere?
The Hill sphere denotes the region surrounding a celestial body within which its gravitational influence dominates over that of another, larger celestial body. Specifically, it represents the boundary at which an object’s gravitational pull on a satellite or smaller object is roughly equal to the gravitational pull of the larger body it orbits.
The Hill sphere’s radius is determined by various factors, including the masses of both bodies and their separation. Objects within this region are more likely to remain gravitationally bound to the primary body, while those outside may be influenced by other celestial bodies. The Hill sphere concept is integral to understanding stable orbits and plays a crucial role in celestial mechanics.
Discovery of Hill Spheres
The discovery of the Hill sphere can be traced back to the pioneering work of George William Hill, an American astronomer who laid the foundation for our understanding of celestial orbits in the late 19th century.
Hill’s keen insights into gravitational interactions led him to formulate the concept in 1878. Building upon the earlier work of Édouard Roche, Hill expanded the understanding of gravitational limits by incorporating the effects of both bodies’ masses and their separation. Roche had already made significant contributions to our comprehension of tidal forces and gravitational limits, setting the stage for Hill to refine and extend these concepts further.
To honor Roche’s original contribution, the Hill sphere is sometimes referred to as the Roche sphere. However this causes confusion, as two other related, but not identical, concepts are also named after him – the Roche limit and the Roche lobe.
By defining a boundary around a celestial body, now known as the Hill sphere, Hill delineated the region where the body’s gravitational influence dominates over that of a larger celestial counterpart. This discovery marked a significant leap in our comprehension of celestial dynamics, providing a framework to analyze the stability of objects in space.
Celestial Dynamics Within Hill Spheres
Within the confines of a Hill sphere, the gravitational influence of a primary body holds sway over orbiting objects. Understanding the dynamics entails examining the delicate interplay between the gravitational forces of the primary body and the intrinsic motion of the orbiting object. This gravitational dominance is particularly pronounced closer to the primary body, gradually diminishing as one moves farther away.
Objects, such as moons or artificial satellites, within the Hill sphere are more likely to be gravitationally bound to the primary body rather than being captured by another celestial body’s gravitational influence. Beyond the Hill sphere, the gravitational influence of other bodies becomes more significant. The concept is particularly relevant in celestial mechanics and the study of satellite dynamics.
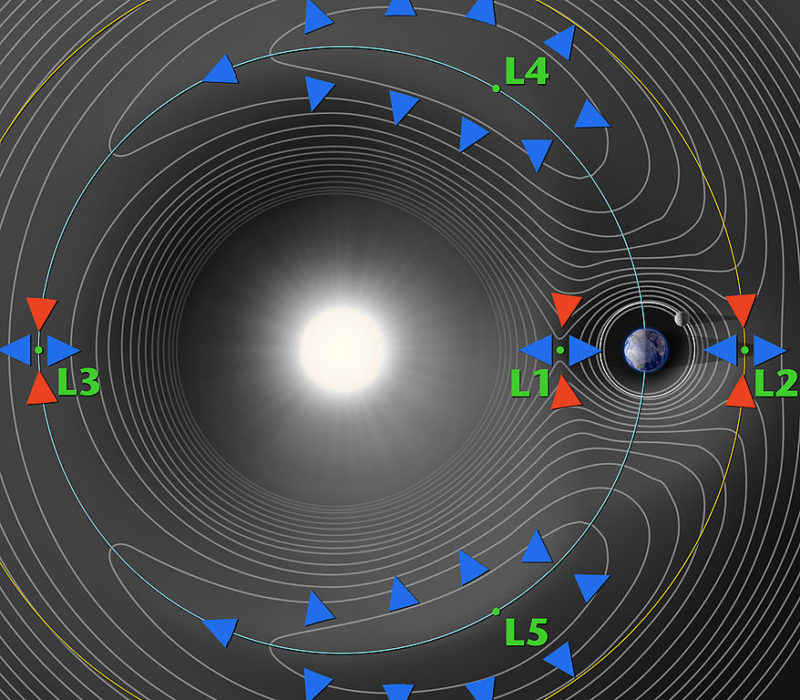
Hill Sphere and Lagrange Points
As we navigate the gravitational intricacies within Hill spheres, an integral aspect of celestial dynamics comes to light—Lagrange points. These specific positions in space, denoted as L1 and L2, represent locations where the gravitational forces between two large celestial bodies reach an equilibrium with the centrifugal force felt by a smaller object. This delicate balance creates stable regions within the celestial expanse.
Within the context of Hill spheres, the relationship with Lagrange points is particularly noteworthy. The Hill sphere, extending between Lagrange points L1 and L2, marks the gravitational domain where the influence of a primary body holds sway. Objects positioned within this region experience a gravitational equilibrium, allowing them to maintain stable orbits relative to the larger celestial bodies.
Beyond the Hill Sphere
In the expanse beyond a Hill Sphere, the gravitational dynamics shift, with external forces assuming prominence. Encounters with other celestial bodies and the persistent gravitational pull of the Sun emerge as pivotal determinants influencing the trajectories of orbiting objects. The transition from the sheltered gravitational confines of the Hill Sphere to the unbounded interplanetary space signifies a critical juncture in the celestial narrative.
Within this dynamic expanse, the concept of gravitational capture becomes salient. Objects once subject to the gravitational influence of a specific celestial body may undergo capture by the gravitational field of another. This intricate gravitational interplay across vast cosmic distances introduces layers of complexity to the celestial mechanics governing the trajectories of planets, moons, and other celestial entities.
Practical Applications
The gravitational dynamics within Hill spheres hold significant relevance in various practical scenarios, influencing satellite orbits, mission planning, and our understanding of celestial bodies.
One notable application lies in satellite dynamics. Artificial satellites, crucial for communication, Earth observation, and scientific research, operate within the gravitational confines of Hill spheres. Understanding the stability and gravitational influence within these regions is essential for designing and maintaining satellite orbits. This knowledge ensures the longevity and efficiency of satellite missions.
Moreover, Hill spheres play a vital role in trajectory planning for space missions. Navigating the gravitational domains within Hill spheres allows mission planners to optimize trajectories, conserve fuel, and achieve mission objectives with greater precision.
Hill Spheres of the Solar System
Expanding our scope to the broader cosmic neighborhood, we turn our attention to the specific Hill spheres within our Solar System. Each celestial body, from planets to moons, exhibits a distinct Hill sphere influenced by its mass and proximity to the Sun. Understanding these individual Hill spheres is instrumental in comprehending the overall stability and gravitational dynamics of our solar system.
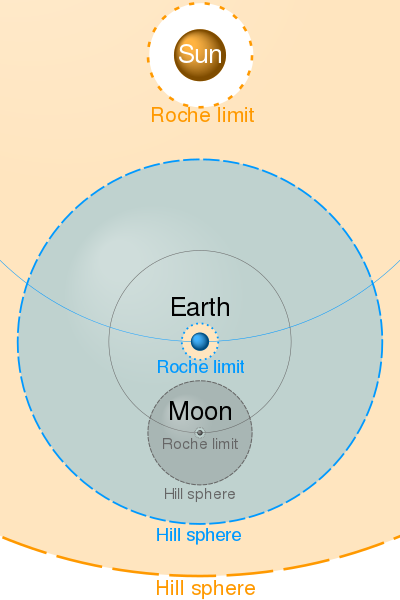
For instance, Earth’s Moon operates within its own Hill sphere, where the gravitational forces of the Moon predominate. Similarly, the Hill Sphere of our planet extends into the space surrounding Earth, influencing the stability of artificial satellites and other orbiting objects. The Earth’s Hill sphere encompasses that of the Moon into what’s called the Earth-Moon system, as illustrated above. Similarly the Sun’s Hill sphere fully encompasses that of the Earth.
Below is a table of the radius of the Hill spheres of some bodies of the Solar System. The values are from the Planetary ephemerides produced by NASA’s Jet Propulsion Laboratory (JPL).
| Celestial Body | Million km | Astronomical units (AU) | Body radii |
|---|---|---|---|
| Mercury | 0.1753 | 0.0012 | 71.9 |
| Venus | 1.0042 | 0.0067 | 165.9 |
| Earth | 1.4714 | 0.0098 | 230.7 |
| Mars | 0.9827 | 0.0066 | 289.3 |
| Ceres | 0.2048 | 0.0014 | 433.0 |
| Jupiter | 50.5736 | 0.3381 | 707.4 |
| Saturn | 61.6340 | 0.4120 | 1022.7 |
| Uranus | 66.7831 | 0.4464 | 2613.1 |
| Neptune | 115.0307 | 0.7689 | 4644.6 |
| Pluto | 5.9921 | 0.0401 | 5048.1 |
| Eris | 8.1176 | 0.0543 | 6979.9 |
Conclusion
Our odyssey through Hill spheres—from their conceptualization by George William Hill to practical applications and cosmic examples—reveals a gravitational narrative that governs our celestial surroundings. Whether within these defined gravitational realms or venturing beyond, the interplay of forces influences satellite missions, trajectory planning, and the captivating dance of celestial bodies. Hill spheres unveil a profound gravitational symphony, shaping the trajectories of cosmic entities in our mesmerizing celestial home.
Would you like to receive similar articles by email?